secretGeek logowiki.secretGeek

What Links Here? (10 articles…)
Outbound Links (47 articles…)

Paradox

logic hilbert catch 22 philosophy grimoire marx heller theseus

I don't want to belong to any club that will accept me as a member.
—Groucho Marx

Shouting loudly into my phone:

"Yes I'm here! Standing right next to your goddam boat! Where the hell are YOU Theseus?"

—https://mastodon.cloud/@secretgeek

What is a paradox? That of course depends on what you mean by "is".

Some paradoxen are statements that are self-contradictory. Some paradoxen are statements that are simultaneously true and untrue. Some paradoxen are statements that use logical methods to arrive at an illogical result. Not all paradoxen are statements.

A paradox can be used to practice critical thinking.

The simplest paradox is the following sentence:

This sentence is false.

Think about it... think about it... got it yet? Oh. Keep thinking...

Yes, really, if you don't get the inherent joy of that one you can just give up now and stop reading this whole site.

How about this.

People complain that Jack lies the whole time, and it's true: he has always lied in the past. Then, one day Jack himself announces: "I always lie". Does Jack always lie?

Some Simple Paradoxen

Paradox Club

Welcome to Paradox club. The first rule of Paradox Club is not the first rule of Paradox Club.

The Rules

The rules are: there are no rules

— Transvision Vamp: Revolution Baby

The rules are there ain't no rules.

— Leo, the head of the Scorpions gang to Travolta's Danny Zuko before their high stakes drag race

Great Paradoxen of the Ancient World

Zeno! Zeno! Good old Zeno. Where was I? You'd set out for a walk with Zeno, start talking and before you knew it you'd be all wrapped up in illogical conundrums that made your head hurt and had you regretting the mead you'd imbibed over lunch.

What were Zeno's Paradoxen?

There's a set of them, and they all involve dividing something by smaller and smaller amounts. If you've ever had to work out your tax, you're probably familiar with the whole idea already. Here's the first one, as retold by our old mate Plato:

In a race, the quickest runner can never overtake the slowest, since the pursuer must first reach the point whence the pursued started, so that the slower must always hold a lead

Okay... so let's slow things down quite a bit, and try to tell that one in a few more words than Plato gave it.

There's a hare and there's a tortoise. In a fair race, the hare would of course be much faster than the tortoise.

Now in this particular race, we give the tortoise a bit of a head start, until, at time T = 0 the hare is allowed to start running.

Some small amount of time later, at time T = 1, the hare will indubitably have reached the position that the turtle was at, at time T = 0. By this time, the turtle of course has moved on, and reached a new location. So the hare has not yet reached the turtle.

And thus:

Some smaller amount of time after that, at time T = n+1, the hare will indubitably have reached the position that the turtle was at, at time T = n. By this time, the turtle of course has moved on, and reached a new location. So the hare has not yet reached the turtle.

Goto "And thus", ad infinitum. The hare can never reach the turtle.

Understood? The hare, who is faster than the turtle, can never, in an infinity of moments, ever reach our turtle friend.

Never give anyone a head start.

The Ship of Theseus

Imagine there is a splendid ship of some historical significance, the Ship of Theseus, and that it is still in service.

Inevitably, a few of the wooden boards of the ship begin to wear out, so these are removed to a museum, and replaced with wooden boards of identical design, constructed using the same materials as the original, and the same ship building processes.

Inevitably, more wooden boards begin to wear out, even the mast, and on each occasion the parts are similarly removed to a museum and replaced.

Eventually there are two Ships of Theseus: the one that is still riding the seas, and the one, fully reconstructed, in the museum. Which is the real Ship of Theseus?

Similarly consider the case of George Washington's axe. The very axe that was owned by George Washington himself, and it is still in use today. The head of the axe has been replaced a few times, as has the shaft, but it's still the same axe.

These are my great, great, grandfather’s apps! He wrote them hundreds of years ago, in something called “VB” and we’ve kept them running ever since.
 Sure we had to rewrite the front end, twice, and the back end, three times, but these are my great, great, grandfather’s apps.

—secretGeek on mastodon

Bertrand Russell, Making Life Impossible for Frege Since 1902!

A paradox that had tremendous impact on the world is "Russell's Paradox", so named because it was a paradox put forward by Bertrand Russell, in a letter to Gottlob Frege. It turned the tables completely on the foundations of mathematics, and, in time, brought about revolutions in the worlds of Logic and Philosophy including (perhaps) the invention of the computer. And it really burnt up our pal Gottlob.

Russell's paradox is all about sets. Specifically it is about sets that are not members of themselves.

First, you have to consider that a set might contain a set. What if you had a set of your favorite sets. That would be a set of sets.

Next, you have to consider that a set that contains sets, might contain itself. If the set of your favorite sets, was itself, one of your favorite sets, then the set of your favorite sets would contain itself: the set of your favorite sets.

Now consider that some sets that contain sets do not contain themselves. For example, the set of your least favorite sets might contain: "the set of sea-lions", "the set of sloths" and so on, but not contain "the set of our least favorite sets", as you might be rather fond of that set.

Once you've got all of those concepts under your belt you're ready to move onto Russell's paradox.

What sets do we find in "the set of all sets that do not contain themselves"? Let's call this set R.

Does R contain R? If R doesn't contain R, then we can say it is a set of sets which doesn't contain itself. Therefore, for completeness, it should contain R. But if it does contain R, then it now contains itself, in which case it shouldn't contain R. But if it doesn't contain R, it should. So if it does it doesn't, and if it doesn't then it does.

When pondering R we either continue down a path of unbounded recursion or we sit down and feel rather dizzy.

Russell packed all of this into a short note he sent to Gottlob Frege, and Frege was most vexed! Our poor buddy Frege had just been putting the finishing touches on the second edition of his Magnum Opus, the Grundgesetze der Arithmetik, II, or "Basic Laws of Arithmetic", a big bumper edition in which Frege attempted to completely define all of mathematics in terms of sets, and reduce sets down to predicate logic. Thus reducing all of maths into a branch of logic.

To say Russell's letter was a blow to Frege would be an understatement. He tried to put on a brave face. He wrote a hasty Appendix to Vol. 2, beginning with this beguiling admission:

Hardly anything more unfortunate can befall a scientific writer than to have one of the foundations of his edifice shaken after the work is finished. This was the position I was placed in by a letter of Mr. Bertrand Russell, just when the printing of this volume was nearing its completion.

In the remainder of the appendix he attempted to derive a resolution to the contradiction by modifying one of his Basic Laws, though it was a poor attempt at best.

Hilbert's Hotel

There's this geezer Hilbert and he has this hotel (he has a curve too, but that is whole other story). Anyway, the Hotel is quite a place.

I'm pretty sure that the song Hotel California is about Hilbert's Hotel. Particularly the chorus where they sing "There's plenty of room at the Hotel California" because let me tell you, there are an infinite number of rooms at Hilbert's. More on this there.

Catch-22

You can't write anything about "Catch-22" that hasn't already been written. It's a novel, from the distant past, set in a time known as The World War II, when people everywhere were trying to kill other people.

The main character is a man called John Yossarian. He's a captain in a US Air Force squadron, in charge of a B-25 bomber. He doesn't want to fly any more missions, and is talking to a Doctor, Doc Daneeka, to see if he can get out of flying.

There was only one catch and that was Catch-22, which specified that a concern for one's safety in the face of dangers that were real and immediate was the process of a rational mind. Orr was crazy and could be grounded. All he had to do was ask; and as soon as he did, he would no longer be crazy and would have to fly more missions. Orr would be crazy to fly more missions and sane if he didn't, but if he was sane he had to fly them. If he flew them he was crazy and didn't have to; but if he didn't want to he was sane and had to. Yossarian was moved very deeply by the absolute simplicity of this clause of Catch-22 and let out a respectful whistle.

"That's some catch, that Catch-22," he observed.

"It's the best there is," Doc Daneeka agreed." —Joseph Heller, Catch-22

Visual Paradox

A paradox can also be presented in a visual manner, such as the paradoxical shapes popularized by MC Escher

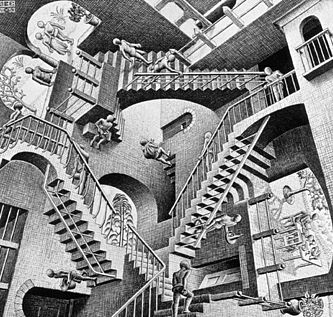
escher_stairs.png

"Mr Escher's office please"
"Up the stairs, keep turning right"
—@MooseAllain

The Paradox of Tolerance

George Carlin expressed the paradox of tolerance in a quintessential way:

So I say, "Live and let live." That's my motto. "Live and let live." Anyone who can't go along with that? Take him outside and shoot the motherfucker.

Temporal Paradox

(Full article: see Temporal Paradox)

A paradox can be a causal conundrum that arises in a time-travelling tale.

For example, what would happen if you went back in time, encountered a young man, killed him, and subsequently learned that he was your own grandfather (your mother's father), on his way to meet your young grandmother for the first time? If he'd never met her, then your mother could never have been born, and neither could you. In which case you never would've had a chance to zip back in time, kill the grandfather.... in which case the grandfather would've not been killed, thus gone on to father the child who ultimately mothered you, thus giving you the chance to go back and kill the grandfather, thus... and thus... etc., in infinite regress. Many many time travel stories derive all of their pleasure from creating, and somehow resolving, a complex paradox.

Jevon's Paradox

"If you increase the efficiency with which a resource is used, people will just use more of it."

This is a nasty tricky sort of a problem, and understanding it is a key part of system thinking.

In 1865, the English economist William Stanley Jevons observed that technological improvements that increased the efficiency of coal-use led to the increased consumption of coal in a wide range of industries. He argued that, contrary to common intuition, technological progress could not be relied upon to reduce fuel consumption.

wikipedia: Jevon's Paradox

Tweets

The Brain in a vat, or jar, argument (or paradox)

How can/would a brain in a vat, or jar, know that it was/wasn't a brain in a vat, or jar? And by extension, how do you know that you're not a brain in a vat, or jar?

Consider the possibility that a demented scientist, alien entity, or hyper-malevolent thinking machine, has removed a human's brain from their skull, suspended it in a gelatenous life-sustaining substance, attached all the necessary wires to connect it to a supercomputer that emits electrochemical impulses which perfectly mimic the input a healthy brain would otherwise receive. The result is that the person now believes themselves to be living in a real world, when indeed it is a mere simulation.

How can they know, ever, that they are (or are not) in a simulation?

There are various solutions to the puzzle. I thought maybe the brain might want to try wiggling back and forth a bit to see if the vat, or jar, rattles its way off the desk and smashes on the floor, but apparently the brain does not have any tiny muscles with which to do this wiggling. Apparently there is a razor from a man called Occam that is one of the primary defeaters of the Brain in a vat, or jar, conundrum.

My best solution, is, I think, quite good:

My resolution to this one is simple. A brain being kept alive in a vat (or jar) sounds like a form of healthcare, and you’d soon learn about it when the demands for payment start gushing in. But I’m sure wiser brains in bigger vats (or jars) have better answers.

— @secretgeek@mastodon.cloud

Newcomb's Paadox

Multiple Choice: If you choose an answer to this question at random, what is the chance you will be correct?

  1. 25%
  2. 50%
  3. 60%
  4. 25%

You don't even know the full question until you've seen the possible answers. Therefore the question depends on the answer. And we know (as always) that the answer depends on the question. Therefore we have a deadlock, a live lock, an Unbounded Recursion.

A Chicken and Egg Problem

The paradox of the chicken and the egg goes like this:

Which came first the chicken or the egg?

If the chicken came first, then where did it come from? From an egg! So the egg came first. But if the egg came first, who laid it? A chicken! So the chicken must have come first. But, if the chicken came first, then where did it come from? From an egg! So the egg came first. But if the egg came first, who laid it? A chicken! So the chicken must have come first. But, if the chicken came first, then where did it come from? From an egg! So the egg came first. But if the egg came first, who laid it? A chicken! So the chicken must have come first. But, if the chicken came first, then where did it come from? From an egg! So the egg came first. But if the egg came first, who laid it? A chicken! So the chicken must have come first. But, and so on.

They co-evolved. But eggs existed long before chickens, so the answer is "the egg." Here's a scientific illustration that supports this hypothesis.

a chicken and egg problem

See Also